top of page
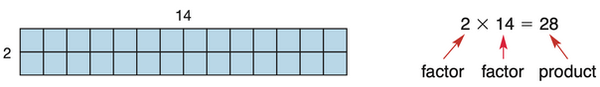
Factors & Products

MULTIPLES

PRIME & COMPOSITE
2 x 14 = 28
Factor
Factor
Product
Prime Number: A number with exactly 2 factors: 1, and itself
Example) 23
1
23
23 can only be divided by 1, and itself (23); therefore only has two factors and thus is a prime number
Composite Number: A number with more than 2 factors
Example) 6
2
3
6 can be divided by 2, 3, 6, and 1 therefore has more than two factors and thus is a composite number.
6
1

FACTORS
Factors
Numbers multiplied to form a product are factors of that product
2 and 14 are factors of 24
Prime Numbers: A number with exactly two factors:
1 and itself
Example: 23 has only two factors; 1 and 23
Composite Numbers: A number with more than two factors:
Example: 24 has multiple factors:
Factors of 24: 1, 2, 3, 4, 6, 8, 12, 24
4 x 6 = 24
2 x 12 = 24
24 x 1 = 24
8 x 3 = 24
Factor Trees
6
4
2
3
2
24
2
8
3
2
4
1
3
24
12
2
2
6
2
1
24
1
2
3
Circle the unique factors: (6, 4) (2, 3) (8, 3) (12, 2) (24, 1)
Practice
Practice PDFs available for download:

PRIME FACTORIZATION
Prime factorization allows us to factor a number and find its prime factors.
Example: 24 has multiple factors:
Factors of 24: 1, 2, 3, 4, 6, 8, 12, 24
4 x 6 = 24
2 x 12 = 24
24 x 1 = 24
8 x 3 = 24
Example) 24
2
12
2
6
2
3
Circle the prime factors: 2, & 3
3
GREATEST COMMON FACTOR (GCF)
GCF using Prime Factorization:
Example) Find the GCF of 138 and 198
138
6
23
2
3
2
198
18
11
6
3
3
Step 1) Find prime factors of each number
Step 2) Find which prime factors are in common
138 Prime Factors: 2 x 3 x 23
198 Prime Factors: 2 x 3 x 11
2
GCF: 2 x 3 = 6
Step 3) The GCF is the product of these common factors

LOWEST COMMON MULTIPLE (LCM)
LCM using Prime Factorization:
Example) Find the LCM of 18, 20, 30
18
6
3
2
3
30
10
3
5
2
20
10
2
5
2
Step 1) Find prime factors of each number
18 Prime Factors: 2 x 3
20 Prime Factors: 2 x 5
Step 2) Of each set, circle which factor is the largest (numbers with higher exponents come first)
30 Prime Factors: 2 x 3 x 5
2
2
2
2
LCM: 3 x 2 x 5 = 180
Step 3) The LCM is the product of these largest factors
bottom of page


